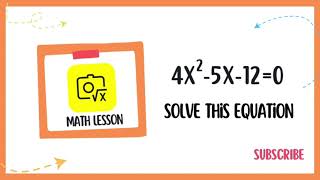
Welcome to this article, where we will delve into the fascinating world of quadratic equations. Specifically, we will focus on solving the equation 4x^2 – 5x – 12 = 0. Quadratic equations are an essential part of algebra and hold significant importance in various fields of mathematics and science. Throughout this article, we will explore the steps involved in solving this equation, discuss its applications, and provide examples to enhance our understanding.
KEY POINTS
What is a Quadratic Equation?
Quadratic equations are polynomial equations of the second degree, which means they involve variables raised to the power of two. In the general form, a quadratic equation can be represented as ax^2 + bx + c = 0, where a, b, and c are coefficients, and x is the variable we are solving for.
Understanding the General Form
In our specific equation, 4x^2 – 5x – 12 = 0, the coefficients are a = 4, b = -5, and c = -12. By rearranging the equation, we can see that it follows the general form of a quadratic equation.
Characteristics of Quadratic Equations
Quadratic equations exhibit certain characteristics that are crucial to their study and solution. These include having a parabolic shape, a vertex that represents the minimum or maximum point, and symmetrical properties. Understanding these characteristics helps us gain insights into their behavior and practical applications.
Solving the Quadratic Equation: 4x^2 – 5x – 12 = 0
To solve the equation 4x^2 – 5x – 12 = 0, we can employ different methods, such as the factoring method or the quadratic formula. Let’s explore each of these techniques.
Factoring Method
The factoring method involves breaking down the quadratic equation into its factors, which can be solved individually. However, this method works only when the equation is factorable.
To solve 4x^2 – 5x – 12 = 0 using the factoring method, we need to find two binomials that multiply together to give us the quadratic equation. In this case, the factored form would be (2x + 3)(2x – 4) = 0.
By equating each factor to zero, we obtain two equations: 2x + 3 = 0 and 2x – 4 = 0. Solving these equations gives us the values of x. Therefore, x = -3/2 and x = 2 are the solutions to the equation.
Quadratic Formula
The quadratic formula provides an alternative method to solve quadratic equations. It is a powerful tool that guarantees finding the solutions, even when the equation is not easily factorable.
For the equation 4x^2 – 5x – 12 = 0, we can use the quadratic formula:
x = (-b ± √(b^2 – 4ac)) / 2a
Plugging in the values a = 4, b = -5, and c = -12 into the formula, we can calculate the solutions for x. After performing the necessary calculations, we find that x = -3/2 and x = 2.
Also Read:
Real-World Applications of Quadratic Equations
Quadratic equations have numerous applications in various fields, including physics, engineering, and economics. Let’s explore a couple of real-world scenarios where quadratic equations are commonly employed.
Projectile Motion
Quadratic equations play a fundamental role in understanding the motion of projectiles. Whether it’s a baseball being thrown, a bullet being fired, or a rocket being launched, the path of these objects can be modeled using quadratic equations. By considering the initial velocity, angle of projection, and the forces acting on the object, we can accurately predict its trajectory.
Engineering and Physics
In engineering and physics, quadratic equations are vital for solving problems related to optimization, motion, and equilibrium. From designing bridges and analyzing structural stability to calculating the behavior of electrical circuits, quadratic equations provide engineers and physicists with powerful mathematical tools to tackle complex problems.
Examples of Solving 4x^2 – 5x – 12 = 0
To solidify our understanding, let’s work through a couple of examples demonstrating how to solve the quadratic equation 4x^2 – 5x – 12 = 0 using both the factoring method and the quadratic formula.
Example 1: Factoring Method
Let’s factorize 4x^2 – 5x – 12 = 0:
(2x + 3)(2x – 4) = 0
By equating each factor to zero, we obtain:
2x + 3 = 0 –> x = -3/2
2x – 4 = 0 –> x = 2
Therefore, the solutions to the equation are x = -3/2 and x = 2.
Example 2: Quadratic Formula
Using the quadratic formula, we can solve 4x^2 – 5x – 12 = 0:
x = (-(-5) ± √((-5)^2 – 4 * 4 * (-12))) / (2 * 4)
After performing the calculations, we find the solutions to be x = -3/2 and x = 2.
Conclusion
In conclusion, we have explored the quadratic equation 4x^2 – 5x – 12 = 0 and examined different methods to solve it. By using the factoring method or the quadratic formula, we can find the values of x that satisfy the equation. Furthermore, we have discovered the real-world applications of quadratic equations in fields such as projectile motion, engineering, and physics. Understanding quadratic equations is essential for solving a wide range of mathematical problems and provides valuable insights into the behavior of various phenomena.